4-manifolds in 7-space

This page has been accepted for publication in the Bulletin of the Manifold Atlas.

The user responsible for this page is Askopenkov. No other user may edit this page at present.

1 Introduction

Most of this page is intended not only for specialists in embeddings, but also for mathematician from other areas who want to apply or to learn the theory of embeddings.

Basic results on embeddings of closed connected 4-manifolds in 7-space are particular cases of results on embeddings of $\newcommand{\Z}{\mathbb{Z}} \newcommand{\R}{\mathbb{R}} \newcommand{\C}{\mathbb{C}} \newcommand{\N}{\mathbb{N}} \newcommand{\Q}{\mathbb{Q}} \newcommand{\F}{\mathbb{F}} \newcommand{\bZ}{\mathbb{Z}} \newcommand{\bR}{\mathbb{R}} \newcommand{\bC}{\mathbb{C}} \newcommand{\bH}{\mathbb{H}} \newcommand{\bQ}{\mathbb{Q}} \newcommand{\bF}{\mathbb{F}} \newcommand{\bN}{\mathbb{N}} \DeclareMathOperator\id{id} % identity map \DeclareMathOperator\Sq{Sq} % Steenrod squares \DeclareMathOperator\Homeo{Homeo} % group of homeomorphisms of a topoloical space \DeclareMathOperator\Diff{Diff} % group of diffeomorphisms of a smooth manifold \DeclareMathOperator\SDiff{SDiff} % diffeomorphism under some constraint \DeclareMathOperator\Hom{Hom} % homomrphism group \DeclareMathOperator\End{End} % endomorphism group \DeclareMathOperator\Aut{Aut} % automorphism group \DeclareMathOperator\Inn{Inn} % inner automorphisms \DeclareMathOperator\Out{Out} % outer automorphism group \DeclareMathOperator\vol{vol} % volume \newcommand{\GL}{\text{GL}} % general linear group \newcommand{\SL}{\text{SL}} % special linear group \newcommand{\SO}{\text{SO}} % special orthogonal group \newcommand{\O}{\text{O}} % orthogonal group \newcommand{\SU}{\text{SU}} % special unitary group \newcommand{\Spin}{\text{Spin}} % Spin group \newcommand{\RP}{\Rr\mathrm P} % real projective space \newcommand{\CP}{\Cc\mathrm P} % complex projective space \newcommand{\HP}{\Hh\mathrm P} % quaternionic projective space \newcommand{\Top}{\mathrm{Top}} % topological category \newcommand{\PL}{\mathrm{PL}} % piecewise linear category \newcommand{\Cat}{\mathrm{Cat}} % any category \newcommand{\KS}{\text{KS}} % Kirby-Siebenmann class \newcommand{\Hud}{\text{Hud}} % Hudson torus \newcommand{\Ker}{\text{Ker}} % Kernel \newcommand{\underbar}{\underline} %Classifying Spaces for Families of Subgroups \newcommand{\textup}{\text} \newcommand{\sp}{^}n$-manifolds in $(2n-1)$-space which are discussed in [Skopenkov2016e], [Skopenkov2006, $\S$2.4 `The Whitney invariant']. In this page we concentrate on more advanced classification results peculiar for $n=4$.

For a general introduction to embeddings as well as the notation and conventions used on this page, we refer to [Skopenkov2016c, $\S$1, $\S$3]. Unless specified otherwise, we work in the smooth category. For the definition of the embedded connected sum $\#$ of embeddings of closed connected 4-manifolds $N$ in 7-space and for the corresponding action of the group $E^7_D(S^4)$ on the set $E^7_D(N)$, see e.g. [Skopenkov2016c, $\S$4].

Remark 1.1 (PL and piecewise smooth embeddings). Any smooth manifold has a unique (up to PL homeomorphism) PL structure compatible with the given smooth structure [Milnor&Stasheff1974, Complement, Theorems 10.5 and 10.6]. Since also any PL 4-manifold admits a unique smooth structure [Mandelbaum1980, $\S$1.2], we may consider a smooth 4-manifold as a PL 4-manifold.

A map of a smooth manifold is 'piecewise smooth (PS)' if it is smooth on every simplex of some triangulation of the manifold. Clearly, every smooth or PL map is PS.

For a smooth manifold $N$ let $E^m_{PS}(N)$ be the set of PS embeddings $N\to\R^m$ up to PS isotopy. The forgetful map $E^m_{PL}(N)\to E^m_{PS}(N)$ is 1-1 [Haefliger1967, 2.4]. So a description of $E^m_{PS}(N)$ is equivalent to a description of $E^m_{PL}(N)$.

2 Examples of knotted tori

The Hudson tori $\Hud_{7,4,2}(a):S^2\times S^2\to S^7$ and $\Hud_{7,4,1}(a):S^1\times S^3\to S^7$ are defined for an integer $a$ in Remark 3.5.d of [Skopenkov2016e] or in [Skopenkov2006, Example 2.10].

Define $D^m_+,D^m_-\subset S^m$ by the equations $x_1\ge0$ and $x_1\le0$, respectively.

Example 2.1 (Spinning construction). For an embedding $g:S^3\to D^6$ denote by $Sg$ the embedding

$$\displaystyle S^1\times S^3\xrightarrow{g\times\id S^1} D^6\times S^1\xrightarrow{{\rm i}_{7,1}}S^7.$$

The restriction of $Sg$ to $D^1_+\times S^3$ is isotopic to (the restriction to $D^1_+\times S^3$ of) the standard embedding. We conjecture that if $t:S^3\to D^6$ is the Haefliger trefoil knot [Skopenkov2016t, Example 2.1], then $St$ is not smoothly isotopic to the connected sum of the standard embedding and any embedding $S^4\to S^7$.

The following Examples 2.2 and 2.3 appear in [Skopenkov2002, $\S$6], [Skopenkov2006, $\S$6] but could be known earlier.

Example 2.2. Two embeddings $\tau^1,\tau^2:S^1\times S^3\to S^7$ are defined as compositions

$$\displaystyle S^1\times S^3\xrightarrow{{\rm pr}_2\times t^k} S^3\times S^3\xrightarrow{{\rm i}_{7,3}} S^7,$$

where $k=1,2$ and maps $t^k:S^1\times S^3\to S^3$ are defined below. We shall see that $t^k|_{S^1\times y}$ is an embedding for any $y\in S^3$ and $k=1,2$, hence $\tau^1$ and $\tau^2$ are embeddings.

Define $t^1(s,y):=sy$, where $S^3$ is identified with the set of unit length quaternions and $S^1\subset S^3$ with the set of unit length complex numbers.

Define $t^2(e^{i\theta},y):=\eta(y)\cos\theta+\sin\theta$, where $\eta:S^3\to S^2$ is the Hopf fibration and $S^2$ is identified with the 2-sphere formed by unit length quaternions of the form $ai+bj+ck$.

It would be interesting to know if $\tau^2$ is PS or smoothly isotopic to the Hudson torus $\Hud_{7,4,1}(1)$.

Example 2.2 can be generalized as follows.

Example 2.3. Define a map $\tau \colon \Z^2 \to E^7(S^1 \times S^3)$. Take a smooth map $\alpha:S^3\to V_{4,2}$. Assuming that $V_{4, 2}\subset (\R^4)^2$, we have $\alpha(x) = (\alpha_1(x), \alpha_2(x))$. Define the adjunction map $\R^2 \times S^3 \to \R^4$ by $((s, t), x) \mapsto \alpha_1(x)s + \alpha_2(x)t$. (Assuming that $V_{4, 2}\subset (\R^4)^{\R^2}$, this map is obtained from $\alpha$ by the exponential law.) Denote by $\overline\alpha:S^1\times S^3\to S^3$ the restriction of the adjunction map. We define the embedding $\tau_\alpha$ to be the composition

$$\displaystyle S^1\times S^3 \xrightarrow{\overline\alpha\times{\rm pr}_2} S^3\times S^3 \xrightarrow{{\rm i}_{7,3}} S^7.$$

We define the map $\tau$ by $\tau(l, b):=[\tau_{\alpha}]$, where $\alpha\colon S^3 \to V_{4, 2}$ represents $(l, b) \in \pi_3(V_{4, 2})$ (for the standard identification $\pi_3(V_{4, 2})=\Zz^2$).

Clearly, $[\tau^1]=\tau(1,0)$ and $[\tau^2]=\tau(0,1)$. See a generalization in [Skopenkov2016k].

It would be interesting to know if $\tau(l,b)=\tau(l,b+2l)$ or $[\tau(l,b)]=[\tau(l,b+2l)]\in E_{PS}^7(S^1\times S^3)$ for any $b,l\in\Zz$.

The unpublished papers [Crowley&Skopenkov2016], [Crowley&Skopenkov2016a] prove that

• any PS embedding $S^1\times S^3\to S^7$ represents $[\tau(l,b)]\in E_{PS}^7(S^1\times S^3)$ for some $l,b\in\Z$.

• any smooth embedding $S^1\times S^3\to S^7$ represents $\tau(l,b)\#a$ for some $l,b\in\Z$ and $a\in E^7(S^4)$.

Example 2.4 (The Lambrechts torus). There is an embedding $S^1\times S^3\to S^7$ whose complement is not homotopy equivalent to the complement of the standard embedding.

I learned this simple construction from P. Lambrechts. Take the Hopf fibration $S^3\to S^7\overset{\nu}\to S^4$. Take the Hopf linking $h:S^1\sqcup S^2\to S^4$ [Skopenkov2016h, Example 2.1]. Then

$$\displaystyle \nu^{-1}h(S^1)\cong S^1\times S^3\quad\text{and}\quad S^7-\nu^{-1}h(S^1)\sim \nu^{-1}h(S^2)\cong S^2\times S^3\not\sim S^2\vee S^3\vee S^5\sim S^7-{\rm i}_{7,3}(S^1\times S^3).$$

Figure 1: Three intersecting disks spanning Borromean rings; a torus with a hole spanning one of the rings and disjoint from the spanning disks of the other two rings

The last homotopy equivalence is proved in a more general form $S^m-{\rm i}_{m,q}(S^p\times S^q)\sim S^{m-p-1}\vee S^{m-q-1}\vee S^{m-p-q-1}$ for $m\ge p+q+3$ by induction on $p\ge0$ using the following observation: if $f:N\to S^n$ is an embedding, then $C_{{\rm i}\circ f}\sim\Sigma C_f$.

(I conjecture that $\nu^{-1}h(S^1)=\tau^1(S^1\times S^3)$.)

Figure 2: The Haefliger torus $S^{2k}\times S^{2k}\to S^{6k+1}$

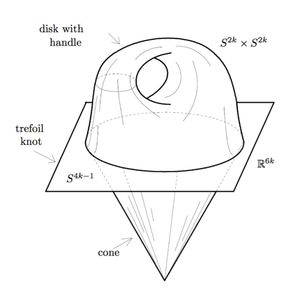
Example 2.5 (the Haefliger torus). There is a PL embedding $S^2\times S^2\to S^7$ which is not PS isotopic to a smooth embedding.

Take the Haefliger trefoil knot $S^3\to S^6$ [Skopenkov2016t, Example 2.1]. Extend it to a PL conical embedding $D^4\to D^7_-$. By [Haefliger1962, $\S$4.2] the trefoil knot also extends to a proper smooth embedding into $D^7_+$ of the punctured torus (or disk with handle), see Figure 1. These two extensions together form the required PL embedding $S^2\times S^2\to S^7$, see Figure 2 for $k=1$. By [Boechat&Haefliger1970, p.165] this PL embedding is not PS isotopic to a smooth embedding.

For a higher-dimensional generalization see [Boechat1971, 6.2].

3 Embeddings of the complex projective plane

Example 3.1 [Boechat&Haefliger1970, p.164]. There is an embedding $\Cc P^2\to\Rr^7$.

Recall that $\Cc P^2_0$ is the mapping cylinder of the Hopf fibration $\eta:S^3\to S^2$. Recall that $S^6=S^2*S^3$. Define an embedding $f:\Cc P^2_0\to S^6$ by $f[(x,t)]:=[(x,\eta(x),t)]$, where $x\in S^3$. In other words, the segment joining $x\in S^3$ and $\eta(x)\in S^2$ is mapped onto the arc in $S^6$ joining $x$ to $\eta(x)$. Clearly, the boundary 3-sphere of $\Cc P^2_0$ is standardly embedded into $S^6$. Hence $f$ extends to an embedding $\Cc P^2\to\Rr^7$.

Alternatively, define an embedding $\Cc P^2\to\Rr^7$ by

$$\displaystyle (x:y:z)\mapsto(x\overline y, y\overline z, z\overline x,2|x|^2+|y|^2),\quad\text{where}\quad |x|^2+|y|^2+|z|^2=1.$$

Theorem 3.2. (a) There is only one embedding $\Cc P^2\to\Rr^7$ up to isotopy and a hyperplane reflection of $\Rr^7$. In other words, there are exactly two isotopy classes of embeddings $\Cc P^2\to\Rr^7$ (differing by composition with a hyperplane reflection of $\Rr^7$).

(b) For any pair of embeddings $f:\Cc P^2\to\Rr^7$ and $g:S^4\to\Rr^7$ the embedding $f\#g$ is isotopic to $f$.

(c) The Boechat-Haefliger invariant (defined below) is an injection $E^7_{PL}(\Cc P^2)\to H_2(\Cc P^2)\cong\Z$ whose image is the set of odd integeres. However, any PL embedding whose Boechat-Haefliger is different from $\pm1$ is not smoothable.

Parts (a) and (b) are proved in [Skopenkov2005, Triviality Theorem (a)] (they also follow by Theorem 5.3 below). Part (c) follows by [Boechat&Haefliger1970, Theorems 1.6 and 2.1] and Corollary 5.6(b) below.

4 The Boechat-Haefliger invariant

We give definitions in more generality because this is natural and is required for 3-manifolds in 6-space [Skopenkov2016t]. Let $N$ be a closed connected orientable $n$-manifold and $f:N\to\Rr^m$ an embedding. Fix an orientation on $N$ and an orientation on $\Rr^m$.

Definition 4.1. The composition

$$\displaystyle H_{s+1}(C_f,\partial)\overset\partial\to H_s(\partial C_f)\overset{\nu_f}\to H_s(N)$$

of the boundary map $\partial$ and the projection $\nu_f$ is an isomorphism. This is well-known, see [Skopenkov2008, $\S$2, the Alexander Duality Lemma]. The inverse $A_{f,s}$ to this composition is `the homology Alexander Duality isomorphism'; it equals to the composition $H_s(N)\to H^{6-s}(C_f)\to H_{s+1}(C_f,\partial)$ of the cohomology Alexander and Poincaré duality isomorphisms.

This is not to be confused with another well-known homology Alexander duality isomorphism $\widehat A_f:H_s(N)\to H_{s+m-n-1}(C)$ [Skopenkov2005, Alexander Duality Lemma 4.6].

Definition 4.2. A `homology Seifert surface' for $f$ is the image $A_{f,n}[N]\in H_{n+1}(C_f,\partial)$ of the fundamental class $[N]$.

Denote by $\cap$ the intersection products $H_{n+1}(C_f,\partial)\times H_{m-n-1}(C_f)\to\Z$ and $H_{n+1}(C_f,\partial)\times H_{n+1}(C_f,\partial)\to H_{2n+2-m}(C_f,\partial)$.

Remark 4.3. Take a small oriented disk $D^{m-n}_f\subset\Rr^m$ whose intersection with $f(N)$ consists of exactly one point of sign $+1$ and such that $\partial D^{m-n}_f\subset\partial C_f$. A homology Seifert surface $Y\in H_{n+1}(C_f,\partial)$ for $f$ is uniquely defined by the condition $Y\cap [\partial D^{m-n}_f]=1$.

Definition 4.4. Define `the Boechat-Haefliger invariant' of $f$

$$\displaystyle \varkappa(f):=A_{f,2n+1-m}^{-1}\left(A_{f,n}[N]\cap A_{f,n}[N]\right)\in H_{2n+1-m}(N).$$

Clearly, a map $\varkappa:E^m(N)\to H_{2n+1-m}(N)$ is well-defined by $\varkappa([f]):=\varkappa(f)$.

Remark 4.5. (a) If $m=2n=6$, then $\varkappa(f)-\varkappa(f_0)=\pm2W(f,f_0)$ for any two embeddings $f,f_0:N\to\Rr^m$ [Skopenkov2008, $\S$2, The Boechat-Haefliger Invariant Lemma]. Here $W$ is the Whitney invariant [Skopenkov2016e, $\S$5], [Skopenkov2006, $\S$2]. We conjecture that this holds when $m-n$ is odd and that $\varkappa(f)=\varkappa(f_0)$ when $m-n$ is even.

(b) Definition 4.4 is equivalent to the original one for $m=2n-1=7$ [Boechat&Haefliger1970] by [Crowley&Skopenkov2008, Section Lemma 3.1]. Earlier notation for $\varkappa(f)$ was $w_f$ [Boechat&Haefliger1970], $BH(f)$ [Skopenkov2005] and $\aleph(f)$ [Crowley&Skopenkov2008].

5 Classification

We use Stiefel-Whitney characteristic classes $w_2$ and (for non-orientable 4-manifolds) $\overline w_3$.

Theorem 5.1. (a) Any closed orientable 4-manifold embeds into $\Rr^7$.

(b) A closed 4-manifold $N$ embeds into $\Rr^7$ if and only if $\overline w_3(N)=0$.

The PL version of (a) was proved in [Hirsch1965]. It was noticed in [Fuquan1994, p. 447] that the smooth version of (a) easily follows from Theorem 5.3.a below by [Donaldson1987]. (The smooth version of (a) also follows from (b) because $\overline w_3=0$ for orientable 4-manifolds [Massey1960].) The smooth version of (b) is [Fuquan1994, Main Theorem A]. The PL version of (b) follows from the smooth version by the second paragraph of Remark 1.1. A simpler proof of the PL versions of (b) is given as the proof of [Skopenkov1997, Corollary 1.3.a] (for specialists recall that $\overline w_3(N)=0\Leftrightarrow\overline W_3(N)=0$ for a closed 4-manifold $N$).

Any compact connected nonclosed 4-manifold embeds into $\Rr^7$. This follows by taking a 3-spine $K$ of $N$, bringing a map $N\to\R^7$ to general position on $K$ and restricting the obtained map to sufficiently thin neighborhood of $K$ in $N$; this neighborhood is homeomorphic to $N$.

For the classical classification in the PL category which uses the assumption $H_1(N)=0$ see [Skopenkov2016e], [Skopenkov2006, Theorem 2.13].

Theorem 5.2. There is an isomorphism $E^7_D(S^4)\cong\Zz_{12}$.

This is stated in [Haefliger1966, the last line] and follows by [Haefliger1966, 4.11] together with well-known fact $\pi_5(G,O)=0$ [Skopenkov2005, Lemma 3.1]. For alternative proofs see [Skopenkov2005, $\S$3, $\S$4] and [Crowley&Skopenkov2008, Corollary 1.2.a].

Let $N$ be a closed connected oriented 4-manifold.

Theorem 5.3. (a) [Boechat&Haefliger1970, Theorems 1.6 and 2.1] The image ${\rm im}\varkappa$ of the Boéchat-Haefliger invariant

$$\displaystyle \varkappa:E^7_D(N)\to H_2(N)$$

$$\displaystyle \text{is}\qquad \{u\in H_2(N)\ |\ u\equiv PDw_2(N)\mod2,\ u\cap u=\sigma(N)\}.$$

(b) [Crowley&Skopenkov2008, Theorem 1.1] If $H_1(N)=0$, then for any $u\in{\rm im}\varkappa$ there is an injective invariant called the Kreck invariant,

$$\displaystyle \eta_u:\varkappa^{-1}(u)\to\Zz_{\gcd(u,24)}$$

whose image is the subset of even elements.

Here

• $PD:H^2(N)\to H_2(N)$ is Poincaré isomorphism.

• $\cap:H_2(N)\times H_2(N)\to\Z$ is the intersection form and $\sigma(N)$ its signature.

• $\gcd(u,24)$ is the maximal integer $k$ such that both $u\in H_2(N)$ and 24 are divisible by $k$.

• $\eta_u$ is defined in [Crowley&Skopenkov2008, $\S$2].

Thus $\eta_u$ is surjective if $u$ is not divisible by 2. Note that $u\in{\rm im}\varkappa$ is divisible by 2 (for some $u$ or, equivalently, for any $u$) if and only if $N$ is spin.

If $H_1(N)=0$, then all isotopy classes of embeddings $N\to\Rr^6$ can be constructed from a certain given embedding using unlinked and linked embedded connected sum with embeddings $S^4\to\Rr^7$ [Skopenkov2016c, $\S$4], [Skopenkov2016e, $\S$3].

For a classification when $H_1(N)\ne0$ see [Crowley&Skopenkov2016] and unpublished paper [Crowley&Skopenkov2016a].

Corollary 5.4 ([Crowley&Skopenkov2008, Corollary 1.2]). (a) There are exactly twelve isotopy classes of embeddings $N\to\Rr^7$ if $N$ is an integral homology 4-sphere (cf. Theorem 5.2).

(b) Identify $H_2(S^2\times S^2) = \Z^2$ using the standard basis. For any integer $u$ there are exactly $\gcd(u,12)$ isotopy classes of embeddings $f:S^2\times S^2\to\Rr^7$ with $\varkappa(f)=(2u,0)$, and the same holds for those with $\varkappa(f)=(0,2u)$. Other values of $\Zz^2$ are not in the image of $\varkappa$.

Addendum 5.5 ([Crowley&Skopenkov2008, Addendum 1.3]). If $H_1(N)=0$ and $f:N\to\Rr^7$, $g:S^4\to\Rr^7$ are embeddings, then

$$\displaystyle \varkappa(f\#g)=\varkappa(f)\quad\text{and}\quad\eta_{\varkappa(f)}[f\#g]\equiv\eta_{\varkappa(f)}[f]+\eta_0[g]\mod\gcd(\varkappa(f),24).$$

The following corollary gives examples where the embedded connected sum action of $E^7_D(S^4)$ on $E^7_D(N)$ is trivial and where it is effective.

Corollary 5.6 ([Crowley&Skopenkov2008, Corollary 1.4]). (a) Take an integer $u$ and the Hudson torus $f_u:=\Hud_{7,4,2}(u):S^2\times S^2\to\Rr^7$ defined in Remark 3.5.d of [Skopenkov2016e], [Skopenkov2006, Example 2.10]. If $u=6k\pm1$, then for any embedding $g:S^4\to\Rr^7$ the embedding $f_u\#g$ is isotopic to $f_u$. Moreover, for any integer $u$ the number of isotopy classes of embeddings $f_u\#g$ is $\gcd(u,12)$.

(b) If $H_1(N)=0$ and $\sigma(N)$ is not divisible by the square of an integer $s\ge2$. Then for any pair of embeddings $f:N\to\Rr^7$ and $g:S^4\to\Rr^7$ the embedding $f\#g$ is isotopic to $f$; in other words, $\varkappa$ is injective.

(c) If $H_1(N)=0$ and $f(N)\subset\Rr^6$ for an embedding $f:N\to\Rr^7$, then for every embedding $g:S^4\to\Rr^7$ the embedding $f\#g$ is not isotopic to $f$.

We remark that Corollary 5.6(b) was first proved in [Skopenkov2005, The triviality Theorem 1.1] independently of Theorem 5.3.

6 References

• [Boechat&Haefliger1970] J. Boéchat and A. Haefliger, Plongements différentiables des variétés orientées de dimension $4$ dans $\Rr^7$, (French) Essays on Topology and Related Topics (Mémoires dédiés à Georges de Rham), Springer, New York (1970), 156–166. MR0270384 (42 #5273) Zbl 0199.27003
• [Boechat1971] J. Boéchat, Plongements de variétées différentiables orientées de dimension $4k$ dans $\Rr^{6k+1}$, Comment. Math. Helv. 46 (1971), 141–161. MR0295373 (45 #4439) Zbl 0218.57016
• [Crowley&Skopenkov2008] D. Crowley and A. Skopenkov, A classification of smooth embeddings of 4-manifolds in 7-space, II, Intern. J. Math., 22:6 (2011) 731-757. Available at the arXiv:0808.1795.
• [Crowley&Skopenkov2016] D. Crowley and A. Skopenkov, Embeddings of non-simply-connected 4-manifolds in 7-space, I. Classification modulo knots, Moscow Math. J., 21 (2021), 43--98. arXiv:1611.04738.
• [Crowley&Skopenkov2016a] D. Crowley and A. Skopenkov, Embeddings of non-simply-connected 4-manifolds in 7-space, II. Smooth classification. Proc. A of the Royal Soc. of Edinburgh, to appear. arXiv:1612.04776
• [Donaldson1987] S. K. Donaldson, The orientation of Yang-Mills moduli spaces and $4$-manifold topology, J. Differential Geom. 26 (1987), no.3, 397–428. MR910015 (88j:57020) Zbl 0683.57005
• [Fuquan1994] F. Fuquan, Embedding four manifolds in $\Rr^7$, Topology 33 (1994), 447-454.
• [Haefliger1962] A. Haefliger, Knotted $(4k-1)$-spheres in $6k$-space, Ann. of Math. (2) 75 (1962), 452–466. MR0145539 (26 #3070) Zbl 0105.17407
• [Haefliger1966] A. Haefliger, Differential embeddings of $S^n$ in $S^{n+q}$ for $q>2$, Ann. of Math. (2) 83 (1966), 402–436. MR0202151 (34 #2024) Zbl 0151.32502
• [Haefliger1967] A. Haefliger, Lissage des immersions-I, Topology, 6 (1967) 221--240.
• [Hirsch1965] M. W. Hirsch, On embedding 4-manifolds in $R^7$, Proc. Camb. Phil. Soc. 61 (1965).
• [Mandelbaum1980] R. Mandelbaum, Four-Dimensional Topology: An introduction, Bull. Amer. Math. Soc. (N.S.) 2 (1980) 1-159.
• [Massey1960] W. S. Massey, On the Stiefel--Whitney classes of a manifold, I, Amer. J. Math. 82 (1960), 92-102.
• [Milnor&Stasheff1974] J. W. Milnor and J. D. Stasheff, Characteristic classes, Princeton University Press, Princeton, N. J., 1974. MR0440554 (55 #13428) Zbl 1079.57504
• [Skopenkov1997] A. Skopenkov, On the deleted product criterion for embeddability of manifolds in $\Rr^m$, Comment. Math. Helv. 72 (1997), 543-555.
• [Skopenkov2002] A. Skopenkov, On the Haefliger-Hirsch-Wu invariants for embeddings and immersions., Comment. Math. Helv. 77 (2002), no.1, 78-124. MRMR1898394 (2003c:57023) Zbl 1012.57035
• [Skopenkov2005] A. Skopenkov, A classification of smooth embeddings of 4-manifolds in 7-space, Topol. Appl., 157 (2010) 2094-2110. Available at the arXiv:0512594.
• [Skopenkov2006] A. Skopenkov, Embedding and knotting of manifolds in Euclidean spaces, in: Surveys in Contemporary Mathematics, Ed. N. Young and Y. Choi, London Math. Soc. Lect. Notes, 347 (2008) 248-342. Available at the arXiv:0604045.
• [Skopenkov2008] A. Skopenkov, A classification of smooth embeddings of 3-manifolds in 6-space, Math. Z. 260 (2008), no.3, 647–672. Available at the arXiv:0603429MR2434474 (2010e:57028) Zbl 1167.57013

• [Skopenkov2016c] A. Skopenkov, Embeddings in Euclidean space: an introduction to their classification, to appear to Bull. Man. Atl.
• [Skopenkov2016e] A. Skopenkov, Embeddings just below the stable range: classification, to appear in Bull. Man. Atl.
• [Skopenkov2016h] A. Skopenkov, High codimension links, to appear in Bull. Man. Atl.
• [Skopenkov2016k] A. Skopenkov, Knotted tori, preprint.
• [Skopenkov2016t] A. Skopenkov, 3-manifolds in 6-space, to appear in Boll. Man. Atl.